Understanding Subitizing in Mathematics: Types, Benefits, and Classroom Strategies

Subitizing is a fundamental skill in mathematics that plays a crucial role in developing number sense and fluency. In this article, we’ll explore what subitizing is, the two different types of subitizing, and how teachers can leverage this skill to enhance students’ mathematical understanding.

What is Subitizing?

Subitizing refers to the ability to instantly recognize the number of items in a set without counting them individually. When we subitize, we bypass the need for sequential counting and perceive the quantity at a glance. Subitizing is part of the big idea that numbers tell us how much and how many and help us to quantify collections of objects (McGarvey, 2018).

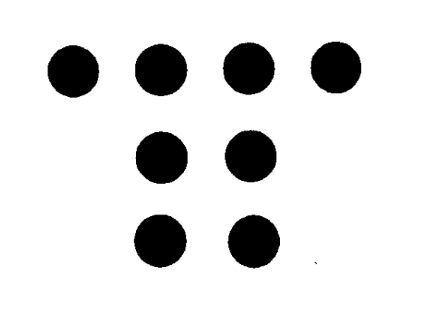
 Let’s delve into the details:

1. Perceptual Subitizing:
   • Definition: Perceptual subitizing involves recognizing small groups of objects (typically up to 5) without counting.
   • Examples:
     • Instantly knowing that there are three dots on a die.
     • Recognizing the number of fingers someone is holding up.
     • Identifying the arrangement of dots on a domino.
   • Benefits: Builds foundational number sense and helps students quickly grasp small quantities.
2. Conceptual Subitizing:
   • Definition: Conceptual subitizing goes beyond recognizing individual groups. It involves combining subgroups to form a whole.
   • Examples:
     • Seeing two groups of three dots and knowing the total is six.
     • Recognizing that 5 can be composed of 3 and 2 or 4 and 1.
     • Understanding that 8 can be split into two groups of four.
   • Benefits: Enhances mental computation, supports addition and subtraction strategies, and fosters deeper understanding of number relationships.

Benefits of Subitizing:

1. Number Sense Development:
   • Subitizing helps students internalize number patterns and relationships.
   • It lays the groundwork for more complex mathematical concepts.
2. Fluency in Basic Operations:
   • Students who can subitize efficiently perform mental calculations more confidently.
   • They can quickly recognize quantities and the part- part whole relationship, thus making addition and subtraction more efficient.
3. Pattern Recognition:
   • Subitizing encourages students to see patterns in numbers and arrangements.
   • This skill extends to recognizing multiples, skip counting, and identifying number bonds.
4. Composing and Decomposing Numbers:
   • Conceptual subitizing enables students to break down numbers into smaller parts.
   • They learn that numbers can be composed of various subgroups.

Classroom Strategies for Subitizing:

1. Use Visual Aids:
   • Display subitizing cards with various arrangements of dots, tally marks, or ten-frames.
   • Encourage students to recognize the quantity instantly.
   • Have students record the groups they see as an expression (e.g. 3+4 is what I see inside of 7).
2. Start Small:
   • Begin with small amounts (under 5) and gradually introduce larger numbers.
   • Help students break larger numbers into smaller groups for quicker recognition. They may even find covering up half the collection with their hand helps them to break the large group into two chunks.
3. Variety of Symbols:
   • Besides dots, use other symbols, images, or objects (e.g., fingers, dice, dominoes).
   • Exposure to diverse representations and arrangements strengthens subitizing skills.
4. Language Matters:
   • Instead of saying “count,” use phrases like “say the number” or “tell me what groups you see” to emphasize instant recognition.
   • Ask students to Find 5 and then ask how many more?
   • Reinforce the concept verbally.

5. Link Subitizing to Basic Facts

• To build conceptual understanding we want students to be able to work from a visual in their mind when adding and subtracting. For example, when I think about 12-9, I think 12 is 4 groups of 3 and 9 is 3 groups of 3 so I will have 1 group of 3 left, thus 12-9=3.
• Subitizing also allows the inverse relationship between addition and subtraction (e.g. 7-3=4 and 4+3=7) to be seen as well as the commutative property of addition (e.g. 3+4 and 4+3). Rather than students memorizing these facts, they have an image to work from.

Remember, subitizing is not just a kindergarten and grade one skill; it’s a foundational building block for mathematical understanding. By incorporating subitizing activities into your classroom from Kindergarten to Grade 10, you empower students to see numbers beyond counting, fostering a deeper appreciation and application for mathematics.

References

McGarvey, L. (2018). Mathematics Learning Progression. Pearson.

https://www.makingnumbersensemakesense.com/post/what-is-subitizing-and-why-is-it-important

https://primarydelightteaching.com/how-to-use-subitizing-cards