Specific Strategies to Differentiate Mathematics Instruction:
Design Open Questions
- Turning around a Question: Instead of giving the question- a teacher provides the answer and asks for the question.
- Asking for Similarities and Differences: Teacher chooses two items (shapes, numbers, graphs etc) and asks students how alike and different they are. There will inevitably be many good answers.
- Replacing a number, share, measurement unit etc with a blank: Teacher replaces a number(s) in a problem with a blank and asks students to choose the number(s) to use. Once students create their responses to the problem, it is valuable to have them share their thinking and compare strategies with their peers (think pair share or whole class discussion).
- Asking for a number sentence: Teacher asks students to create a sentence that includes certain words and numbers.
Parallel Tasks
To meet a variety of needs teachers can decide on a key concept for learning and create 2 parallel tasks that are both focused on that concept, but address students at different levels of mathematical sophistication
Part of the struggle with implementing parallel tasks is maintaining student perseverance throughout the task.
Before you begin planning the tasks– think about how you will maintain student perseverance throughout by considering the following questions:
- What types of misconceptions do you anticipate students will struggle with during the task?
- What types of scaffolding questions can you ask students to help guide their work on this task when they get stuck?
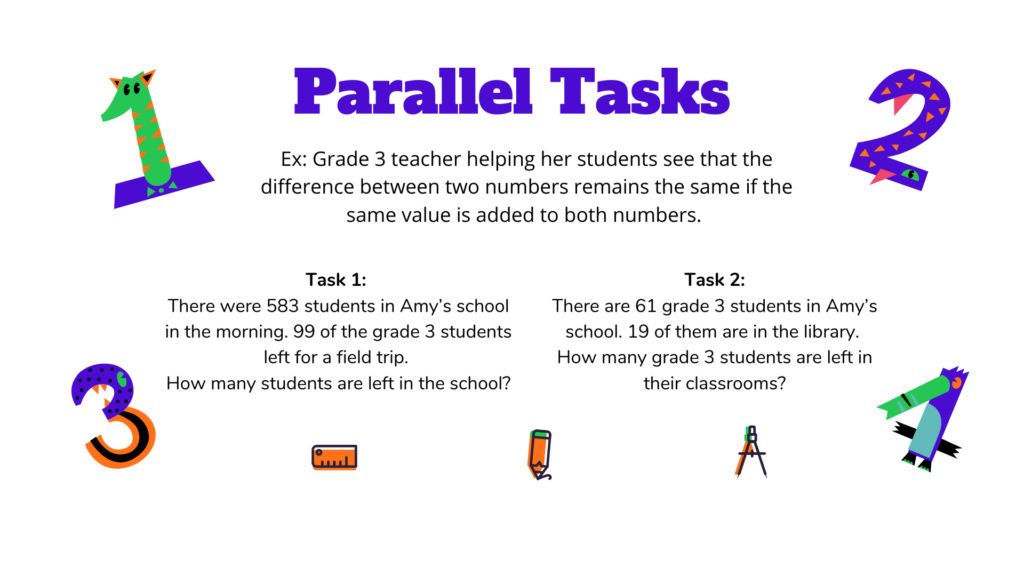
Teachers can assist students in both of the groups attend to the equivalence of the two subtraction situations by asking the following effective questions and prompts:
- How did you know that most of the students were left?
- How did you decide how many were left?
- I notice that “student” solved it by subtracting. Why does subtraction make sense?
- I notice that “student” solved it by adding. Why might adding make sense?
- How would your answer have changed if one more student had left?
- How would your answer have changed if there had been one extra student to start with?
- How would your answer have changed if there was an extra student to start with, but one extra student left?
- Which problem is easier for you to solve?
Parallel Task Examples
References:
More Good Questions: Great Ways to Differentiate Secondary Mathematics Instruction, by Marian Small and Amy Lin, Hawker Brownlow Education, 2011, pp. 1–10.
“Differentiating Mathematics Instruction.” Jessica Kanold-McIntyre, Solution Tree Blog, 26 Feb. 2019, www.solutiontree.com/blog/how-to-differentiate-math-instruction/.
“Differentiating Mathematics Instruction.” The Literacy and Numeracy Secretariat Government of Ontario, Capacity Building Series, Government of Ontario, Sept. 2008, www.edu.gov.on.ca/eng/literacynumeracy/inspire/research/capacityBuilding.html.